We have the group eq frac z nz eq This group is isomorphic to the multiplicative inverse element of the group eqZ_n eq We know that. Z nZ.
45 Questions With Answers In Isomorphism Science Topic
ZnZ is Isomorphic to Zn Recall that denotes the group of integers modulo and denotes the cyclic subgroup of order.
Z isomorphic to nz. But since f1 mZ is an element of ZnZ nf. Announcements Additional assessment materials have been released - more info here. We have already noted that is isomorphic to via an explicit isomorphism.
8272013 Which means we only use units. Then since 1 mZ has order m in ZmZ f1 mZ has order dividing m. ZmZ ZnZ is Z-linear.
According to the German Wikipedia source. Let P be a regular polygon with nvertices. We can define an isomorphism between these groups by the map a n a n Z Note however that a n and a n Z are equal as sets and the group operation is precisely the same so the isomorphism of these two groups is fairly trivial.
ZZmZZnZ ZmnZ be de ned as follows. N pr 2pr p P r N 1 So there is no simple. The isomorphism is Phi z nZ.
L does in fact de ne a map from Hom ZZmZZnZ to ZmnZ. Dihedral groups Fix n2. Check out our guide to uni admission language here.
Prove that Z is isomorphic to nZ where n is not 0. It has the same structure is isomorphic to ZnZ the only difference being one group is written with multiplicative notation and one withadditivenotation. It follows that the corresponding unit group is ZnZ Y Zpe pZ.
Z nZ. The Sun Ze Theorem gives a ring isomorphism ZnZ Y Zpe pZ. In set-builder notation we have that.
For suppose f. Start new discussion reply. Im assuming you mean both are additive groups and that you are allowed to use the distributive law.
By the Lemma this happens if and only if aand nare coprime ie. 4182015 Isomorphism between Zmz Znz to zgcdmn zlcmmn Watch. ZnZ Z n where x nZ x n This means that the map.
See full answer below. Prove That Z Is Isomorphic To NZ Where N Is Not 0. Its worth noting though that they are not isomorphic as rings though as Z has a multiplicative identity element while n Z does not.
The right side is the cartesian product of the rings Zpe pZ meaning that addition and multiplication are carried out componentwise. The proof is the same as for the last claim. 1022017 If you know group theory you could also simply say that n Z and Z are infinite cyclic groups and all infinite cyclic groups are isomorphic.
Answered Oct 3 17 at 1620. Z equiv 0 pmod 2 quad mathrm and quad 1_2 z in mathbb Z. On The Ring of Z2Z page we defined to be the following set of sets.
Suppose p is an odd prime dividing n and 4 nThenZnZ is not cyclic. 4272015 On the other hand the elements of Z n Z can be written as cosets of the form a n Z. Now ZnZ is isomorphic to a direct product of groups including ZpepZ and Z2e2Zwithe 2 2.
1 The set denotes the set of integers such that and the set denotes the set of integers such that. A2ZnZ in which case the map x axis also invertible with inverse 1 x a 1 x. Moreover by Proposition 223 ZnZ is isomorphic to Z n as a group with addition and an explicit isomorphism is given by the map.
We will now prove this fact against using The First Group Isomorphism Theorem. You can also show it is a homomorphism because of the distributive law. ZnZ f0 nZ1 nZn 1 nZgas a set.
This problem has been solved. 2 begin align quad 0_2 z in mathbb Z. Again both groups have even order and so the direct product cant be cyclic.
It is obviously bijective. And Z mZ. The Unit Group of ZnZ Consider a nonunit positive integer n Y pe p 1.
ZnZ is Z-linear and if f1 mZ k nZ then Lf k mnZ. ZnZ Z n given by is a well-de ned b bijective c preserves group operation addition that is.
Pdf On The Structure Of Quaternion Rings Over Mathbb Z N Mathbb Z
Https Www Math Arizona Edu Ura Reports 063 Mcmurdie Christopher Final Pdf
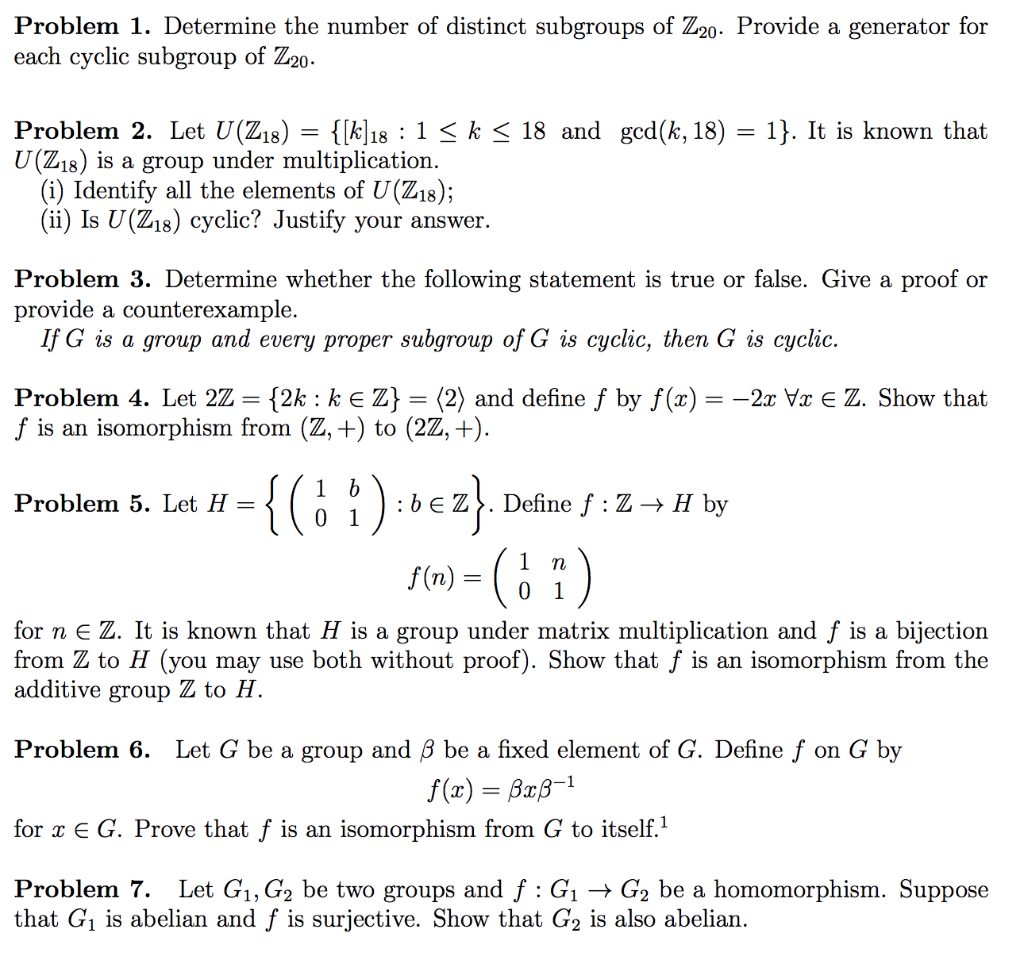Solved Problem 1 Determine The Number Of Distinct Subgro Chegg Com
Section 14 Factor Groups Factor Groups From Homomorphisms Theorem Let G G Be A Group Homomorphism With Kernel H Then The Cosets Of H Form A Factor Ppt Download
Http Www Math Wustl Edu Sli Math430 Spring2016 T2s Pdf
Geiaal89npxrkm
Http Btravers Weebly Com Uploads 6 7 2 9 6729909 Section 3 Isomorphic Binary Structures Homework Solutions Pdf
Https Www Math U Bordeaux Fr Couveign Cours Z Pdf
Https Www3 Nd Edu Ajorza Courses 2014f M60210 Notes Lecture6 Pdf
Section 14 Factor Groups Factor Groups From Homomorphisms Theorem Let G G Be A Group Homomorphism With Kernel H Then The Cosets Of H Form A Factor Ppt Download
Basic Algebra
Prove That Mathbb Z Oplus Mathbb Z Langle A 0 Rangle Times Langle 0 B Rangle Is Isomorphic To Mathbb Z A Oplus Mathbb Z B Mathematics Stack Exchange
Http Math Iit Edu Rellis Teaching 430f06 Exam Finalkey Pdf
Phanny S Report Paper Mathematics Rupp
0 0 Order 1 0 1 Order 4 0 2 Order 2 0 3 Order 4 1 0 Order 2 1 1 Order 4 1 2 Order 2 1 3 Order 4 Pdf Free Download
Example Of Factor Quotient Ring Z 4z Youtube
Isomorphism
5 Cyclic Groups
Section 14 Factor Groups Factor Groups From Homomorphisms Theorem Let G G Be A Group Homomorphism With Kernel H Then The Cosets Of H Form A Factor Ppt Download
Post a Comment
Post a Comment